Marking the radius of a circle around its circumference 6 times divides its circumference into 6 equal parts. If we join these points, one to the next, we gain a hexagon (a six-sided polygon). If then we join each of the 6 points on the circumference with the centre, we divide the hexagon into 6 equilateral triangles.
The equilateral triangle (a triangle in which all three sides have the same length) and the hexagon can fill a flat surface without leaving any spaces in-between. The same is true of the square. It is why these geometric figures form the base grids upon which the Cosmatesque, along with many other, ornaments are constructed.
The triad, represented as an equilateral triangle, symbolises the Holy Trinity one God: Father, Son and Holy Spirit. As the Catechism of the Catholic Church explains:
Therefore, each time we draw an equilateral triangle within the context of the Cosmatesque, ornaments of Roman Catholic churches, we are making a reference to the Holy Trinity.
Materials:
compass
30cm ruler
H or 2H pencil
A4 printer paper
card or cutting mat
eraser
Notes:
place the A4 printer paper in landscape position
use radius of approx. 4 cm, but keep in mind that this construction works regardless of the measurement of the radius and can be scaled up or down according to needs
please use the contact button should you have any queries or would like to share your work
1) Place an A4 page of printer paper in landscape position. Find the approximate middle and with radius of approx. 4 cm draw a circle. Mark the circle centre as point Z.
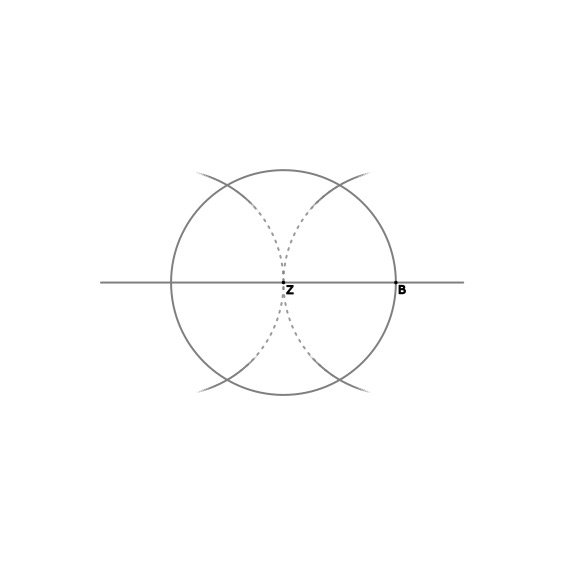
2) Draw a horizontal line across the circle. Allow the line to extend through the circumference of the circle on both left and right. Make sure this line accurately crosses point Z. Mark intersections A and B.
3) Place compass needle in point A and with the original radius, AZ, draw an arch which will cross the circle circumference both above and below the horizontal line. Because it is the intersection points which are important, it is not necessary to draw the arch in full.
4) Mirror the previous action from point B. There should now be two intersections on the circumference above the horizontal line, and two intersections below the horizontal line.
5) Mark these intersections as points C, D, E and F.
6) There should now be six points on the circumference of the circle in equal distance off each other.
7) Join the points in a consecutive manner: A-C-D-B-E-F-A.
8) There should now be a hexagon inscribed into the circle.
9) To gain the 6 equilateral triangles inside the hexagon, and a six-fold-division of the circle: join each of the points C, D, E and F with the centre point Z. The horizontal line already joins points A and B with the centre.
10) Start on a new A4 page. To gain a 12-fold-division of the circle: begin in the same manner, with the A4 printer paper in landscape position and the radius approx. 4 cm. Draw a circle in the approximate middle of the page and mark the centre as point Z.
11) Draw a horizontal line across the circle. Extend the line more than diameter length on each of the side of the circle. Make sure this line accurately crosses point Z. Mark the intersection on the left as point A.
12) Place the compass needle in point A, and with the same radius as previously, AZ, draw a full circle. Mark the point of intersection at the top as point B.
13) With the same radius draw a full circle from point B. Mark intersection point C.
14) With the same radius draw a full circle from point C. Mark intersection point D.
15) With the same radius draw a full circle from point D. Mark intersection point E.
16) With the same radius draw a full circle from point E. Mark intersection point F.
14) With the same radius draw a full circle from point F. The circle should cross point A on the horizon line. There should now be a flower shape with 6 petals in the centre.
15) With diagonal lines join points B with E, and C with F. This will divide the circle into 6 equal parts.
16) The six-fold flower shape is marked with grey. This shape is sometimes referred to amid contemporary geometers as the Flower of Creation.
17) Six larger petal shapes were also created as the circles were added around the circumference of the main circle.
18) Name the tips these points 1, 2, 3, 4, 5 and 6.
19) With a diagonal line join point 1 with 4.
20) With a vertical line join point 2 with 5.
21) With a diagonal line join point 3 with 6.
22) The division of the central circle into 12 equal parts is now complete.